What Is Midrange In Math? A Comprehensive Guide For Everyday Use

Math can sometimes feel like a foreign language, but don't worry—we're here to translate it for you. If you've ever stumbled upon the term "midrange" while diving into math problems, you're not alone. Midrange is one of those terms that sounds fancy but is actually pretty straightforward once you break it down. In this article, we’ll unravel what midrange means in math, how it works, and why it matters. So, grab your favorite snack, and let’s dive in!

Now, before we get into the nitty-gritty, let’s set the scene. Imagine you're working on a project or solving a problem that involves numbers, and you need to find a way to summarize them. That's where midrange comes in handy. It’s like the middle ground in your dataset, giving you a quick snapshot of where your numbers lie. Sounds useful, right?

Whether you're a student trying to ace your math test, a teacher explaining concepts to your class, or just someone curious about numbers, this article has got you covered. We’ll break it down step by step so you can confidently use midrange in your math adventures. Let’s go!

Read also:

Columbus Dispatch Obituaries A Glimpse Into Lives That Matter

Table of Contents

• What is Midrange in Math?
• How to Calculate Midrange
• Real-Life Applications of Midrange
• Midrange vs. Mean: What’s the Difference?
• Advantages and Disadvantages of Using Midrange
• Understanding Biases in Midrange
• Common Mistakes to Avoid
• A Historical Perspective on Midrange
• Tools for Calculating Midrange
• Future Trends in Midrange Analysis

What is Midrange in Math?

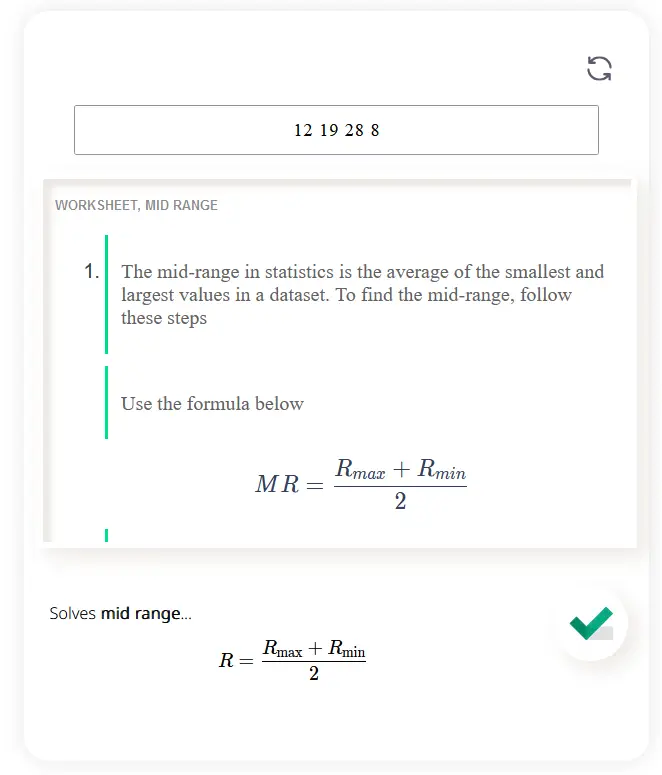
Alright, let’s start with the basics. Midrange in math is essentially the average of the largest and smallest values in a dataset. Think of it as the middle point between the highest and lowest numbers. It’s a quick and easy way to get an idea of where your data is centered. Simple, right?

But here’s the thing—midrange isn’t just about finding the middle. It’s also about understanding the spread of your data. For example, if you’re analyzing test scores, the midrange can give you a rough estimate of the average performance without getting bogged down by all the details.

Breaking Down the Formula

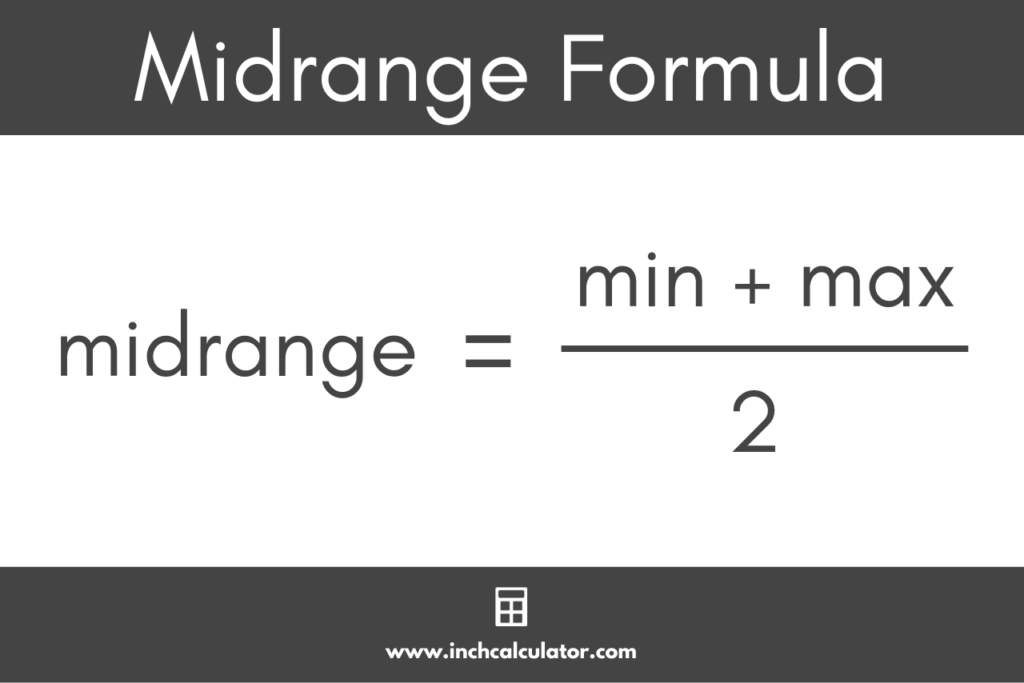
The formula for calculating midrange is as simple as it gets. All you need to do is add the highest and lowest values in your dataset and divide the sum by two. Here's the math behind it:

Midrange = (Maximum Value + Minimum Value) / 2

Let’s say you have a dataset with the following numbers: 5, 10, 15, 20, and 25. The maximum value is 25, and the minimum value is 5. Plug those into the formula:

Read also:

Armslist Tulsa Your Ultimate Guide To Buying Selling And Trading Firearms In The Heart Of Oklahoma

Midrange = (25 + 5) / 2 = 15

Boom! You’ve got your midrange. Easy peasy.

How to Calculate Midrange

Now that we’ve covered the basics, let’s dive deeper into the calculation process. Calculating midrange is a breeze, but there are a few things to keep in mind to ensure accuracy.

Step-by-Step Guide

• Identify the maximum value in your dataset.
• Identify the minimum value in your dataset.
• Add the maximum and minimum values together.
• Divide the sum by two.

Let’s try another example. Imagine you’re analyzing the daily temperatures for a week:

Monday: 20°C, Tuesday: 22°C, Wednesday: 18°C, Thursday: 25°C, Friday: 21°C, Saturday: 19°C, Sunday: 23°C.

The maximum temperature is 25°C, and the minimum temperature is 18°C. Using the formula:

Midrange = (25 + 18) / 2 = 21.5°C

There you go—the midrange temperature for the week is 21.5°C.

Real-Life Applications of Midrange

Midrange isn’t just a theoretical concept—it has real-world applications that can make your life easier. Whether you’re a business owner analyzing sales data or a scientist studying climate patterns, midrange can provide valuable insights.

Business Analytics

In the world of business, midrange can help you quickly assess the performance of your products or services. For example, if you’re tracking monthly sales figures, the midrange can give you a snapshot of your average sales without getting too detailed.

Science and Research

Scientists often use midrange to summarize data in experiments. Let’s say you’re studying the growth of plants under different conditions. The midrange can help you identify the average growth rate without being swayed by outliers.

Midrange vs. Mean: What’s the Difference?

One question that often comes up is, “How does midrange compare to the mean?” Great question! Both midrange and mean are measures of central tendency, but they serve different purposes.

Midrange

Midrange is all about simplicity. It’s quick to calculate and gives you a rough estimate of where your data is centered. However, it doesn’t take into account the distribution of your data, which can be a limitation.

Mean

On the other hand, the mean (or average) considers every value in your dataset. It’s a more comprehensive measure, but it can be skewed by outliers. For example, if you have a dataset with extreme values, the mean might not accurately represent the central tendency.

So, which one should you use? It depends on your goals and the nature of your data. If you need a quick summary, midrange is your go-to. If you want a more detailed analysis, the mean might be better suited.

Advantages and Disadvantages of Using Midrange

Like any statistical measure, midrange has its pros and cons. Let’s break them down:

Advantages

• Easy to calculate
• Quickly provides a central value
• Useful for large datasets where detailed analysis isn’t necessary

Disadvantages

• Doesn’t consider the distribution of data
• Can be misleading if there are significant outliers
• Lacks depth compared to other measures like mean or median

While midrange has its limitations, it’s still a valuable tool when used appropriately. Just remember to consider the context of your data before relying solely on midrange.

Understanding Biases in Midrange

One important aspect to consider is the potential for bias in midrange calculations. Since midrange only takes into account the maximum and minimum values, it can be heavily influenced by outliers. For example, if you have a dataset with a few extreme values, the midrange might not accurately represent the central tendency of your data.

Let’s look at an example. Imagine you’re analyzing the salaries of employees in a company:

$30,000, $35,000, $40,000, $45,000, $50,000, $55,000, $60,000, $1,000,000.

The maximum salary is $1,000,000, and the minimum salary is $30,000. Using the formula:

Midrange = ($1,000,000 + $30,000) / 2 = $515,000

Does $515,000 accurately represent the average salary in this company? Probably not. The extreme value of $1,000,000 skews the midrange, making it less reliable in this case.

Common Mistakes to Avoid

When working with midrange, it’s easy to fall into a few common traps. Here are some mistakes to watch out for:

• Ignoring outliers: As we discussed earlier, outliers can significantly impact your midrange calculation. Make sure to analyze your data carefully before drawing conclusions.
• Using midrange for detailed analysis: Midrange is great for quick summaries, but it’s not suitable for in-depth analysis. If you need more detailed insights, consider using other measures like mean or median.
• Forgetting the context: Always consider the context of your data. What does the midrange tell you about your dataset? Is it relevant to your goals?

By avoiding these mistakes, you can ensure that your midrange calculations are accurate and meaningful.

A Historical Perspective on Midrange

Midrange might seem like a modern concept, but its roots go back centuries. Mathematicians and statisticians have been using measures of central tendency for ages, and midrange is just one of them.

In the early days of statistics, midrange was often used as a quick way to summarize data. As mathematics evolved, more sophisticated measures like mean and median were developed, but midrange remained a useful tool for certain applications.

Today, midrange is still widely used in fields like business, science, and education. Its simplicity and ease of use make it a go-to measure for quick analyses.

Tools for Calculating Midrange

In the age of technology, calculating midrange has never been easier. There are plenty of tools and software available to help you crunch the numbers. Here are a few options:

• Spreadsheets: Programs like Microsoft Excel and Google Sheets make it easy to calculate midrange using built-in functions.
• Statistical Software: Tools like SPSS and R offer advanced features for analyzing data, including midrange calculations.
• Online Calculators: If you prefer a quick and simple solution, there are plenty of online calculators available for free.

Whatever tool you choose, make sure it fits your needs and skill level. Technology can save you a lot of time and effort, but it’s still important to understand the underlying concepts.

Future Trends in Midrange Analysis

As data becomes increasingly important in our daily lives, the role of midrange in analysis is likely to evolve. With advancements in artificial intelligence and machine learning, we may see new ways of using midrange to gain insights from complex datasets.

However, one thing is certain—midrange will continue to be a valuable tool for anyone working with numbers. Its simplicity and versatility make it a staple in the world of mathematics and statistics.

So, whether you’re a student, teacher, or professional, understanding midrange can give you a competitive edge in your field. Stay curious, keep learning, and don’t be afraid to explore new ways of using midrange in your work.

Conclusion

There you have it—a comprehensive guide to midrange in math. From its definition and calculation to its applications and limitations, we’ve covered everything you need to know. Midrange might not be the most sophisticated measure out there, but it’s certainly one of the most useful.

So, the next time you’re faced with a dataset and need a quick summary, remember midrange. It’s your trusty companion in the world of numbers. And who knows? You might just impress your friends and colleagues with your newfound math skills.

Before you go, why not share your thoughts in the comments below? Do you have any tips or tricks for working with midrange? Or maybe you’ve encountered a situation where midrange came in handy. We’d love to hear from you!

And don’t forget to check out our other articles for more math-related goodness. Happy calculating!